what is the result of applying the same glide reflection twice is equivalent​ to?

The operation of a glide reflection: A composite of a reflection across a line and a translation parallel to the line of reflection

Since this footprint trail has glide reflection symmetry, applying the operation of glide reflection will map each left footprint into a right footprint and each right footprint to a left footprint, leading to a final configuration which is indistinguishable from the original.
In ii-dimensional geometry, a glide reflection (or transflection) is a symmetry operation that consists of a reflection over a line and then translation along that line, combined into a single operation. The intermediate stride between reflection and translation tin can expect different from the starting configuration, so objects with glide symmetry are in general, not symmetrical nether reflection alone. In group theory, the glide plane is classified every bit a blazon of opposite isometry of the Euclidean plane.
A single glide is represented as frieze grouping p11g. A glide reflection can exist seen as a limiting rotoreflection, where the rotation becomes a translation. Information technology can also be given a Schoenflies notation every bit Stwo∞, Coxeter notation equally [∞+,2+], and orbifold notation as ∞×.
Description [edit]
The combination of a reflection in a line and a translation in a perpendicular direction is a reflection in a parallel line. However, a glide reflection cannot be reduced similar that. Thus the effect of a reflection combined with whatsoever translation is a glide reflection, with as special case but a reflection. These are the 2 kinds of indirect isometries in 2nd.
For example, there is an isometry consisting of the reflection on the x-centrality, followed by translation of one unit parallel to it. In coordinates, it takes
(x, y) → (x + 1, −y).
This isometry maps the x-axis to itself; whatever other line which is parallel to the 10-axis gets reflected in the 10-axis, so this system of parallel lines is left invariant.
The isometry grouping generated past just a glide reflection is an infinite circadian group.[1]
Combining 2 equal glide reflections gives a pure translation with a translation vector that is twice that of the glide reflection, and then the fifty-fifty powers of the glide reflection course a translation group.
In the case of glide reflection symmetry, the symmetry group of an object contains a glide reflection, and hence the group generated by it. If that is all it contains, this type is frieze grouping p11g.
Case pattern with this symmetry group: ![]()
Frieze group nr. 6 (glide-reflections, translations and rotations) is generated by a glide reflection and a rotation about a point on the line of reflection. It is isomorphic to a semi-direct product of Z and C 2.
Case pattern with this symmetry group: ![]()
A typical case of glide reflection in everyday life would be the track of footprints left in the sand past a person walking on a beach.
For any symmetry group containing some glide reflection symmetry, the translation vector of any glide reflection is one one-half of an chemical element of the translation group. If the translation vector of a glide reflection is itself an chemical element of the translation group, then the corresponding glide reflection symmetry reduces to a combination of reflection symmetry and translational symmetry.
Glide reflection symmetry with respect to 2 parallel lines with the same translation implies that there is also translational symmetry in the direction perpendicular to these lines, with a translation altitude which is twice the distance betwixt glide reflection lines. This corresponds to wallpaper group pg; with additional symmetry information technology occurs besides in pmg, pgg and p4g.
If in that location are also truthful reflection lines in the same management so they are evenly spaced between the glide reflection lines. A glide reflection line parallel to a true reflection line already implies this situation. This corresponds to wallpaper group cm. The translational symmetry is given by oblique translation vectors from ane bespeak on a truthful reflection line to ii points on the next, supporting a rhombus with the true reflection line as one of the diagonals. With boosted symmetry it occurs also in cmm, p3m1, p31m, p4m and p6m.
In 3D the glide reflection is chosen a glide plane. It is a reflection in a aeroplane combined with a translation parallel to the plane.
Wallpaper groups [edit]
In the Euclidean airplane 3 of 17 wallpaper groups require glide reflection generators. p2gg has orthogonal glide reflections and 2-fold rotations. cm has parallel mirrors and glides, and pg has parallel glides. (Glide reflections are shown below as dashed lines)
| Crystallographic proper noun | pgg | cm | pg |
|---|---|---|---|
| Conway name | 22× | *× | ×× |
| Diagram |  |  | 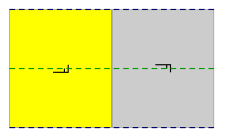 |
| Example |  |  |  |
Glide reflection in nature and games [edit]
Glide symmetry can exist observed in nature amongst certain fossils of the Ediacara biota; the machaeridians; and sure palaeoscolecid worms.[2] It tin also exist seen in many extant groups of sea pens.[3]
Glide reflection is common in Conway's Game of Life when producing Gun (cellular automaton).
Come across also [edit]
For the respective 3D symmetry operations
- Screw axis
- Glide plane
References [edit]
- ^ Martin, George E. (1982). Transformation Geometry: An Introduction to Symmetry. Undergraduate Texts in Mathematics. Springer. p. 64. ISBN9780387906362. .
- ^ Waggoner, B. M. (1996). "Phylogenetic Hypotheses of the Relationships of Arthropods to Precambrian and Cambrian Problematic Fossil Taxa". Systematic Biology. 45 (2): 190–222. doi:10.2307/2413615. JSTOR 2413615.
- ^ Zubi, Teresa (2016-01-02). "Octocorals (Stoloniferans, soft corals, sea fans, gorgonians, sea pens) - Starfish Photos - Achtstrahlige Korallen (Röhrenkorallen, Weichkorallen, Hornkoralllen, Seefedern, Fächerkorallen)". starfish.ch . Retrieved 2016-09-08 .
External links [edit]
- Glide Reflection at cutting-the-knot
Source: https://en.wikipedia.org/wiki/Glide_reflection
0 Response to "what is the result of applying the same glide reflection twice is equivalent​ to?"
ارسال یک نظر